What are Real Numbers? When you are first learning to do math with numbers, you never think about what “kinds” of numbers you use. You just add 2 plus 2, or subtract 10 from 20, and later on begin to multiply (-3) times (-5) and other, more complicated functions. What you don’t even realize is that all the numbers that you are playing with actually belong to a certain set of numbers. And this set of numbers is called Real Numbers. Later on, (much later on,) you might begin to study other sets of numbers, such as the Imaginary Numbers… but that is a much different lesson that we’ll save for another day.
So, just what is a Real Number? Quite simply, a Real Number is any number that you can express in a decimal form. They are the numbers that you use everyday in your math homework, and which also get used in laboratories, offices, and industries. Like I said, you don’t even realize that you’ve been using Real Numbers all along.
The set of Real Numbers is often designated with a rather fancy capital R letter, like this: 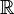
If we think of our set of Real Numbers as being numbers that can be expressed as a decimal, we can similarly think of them as being represented on a number line. As such, it is easy to see that Real Numbers include all the numbers on the number line, whether they are positive, negative, or zero.
Now, if we continue to think of a number line, we can further break down our set of Real Numbers into further categories.
One category is the Rational numbers, which are any numbers that can be expressed as the ratio of two integers where the denominator is not zero (such as 5 (5/1), 2/3 (0.6666…), or 0.87934 which is really 87934/100000…). The whole numbers, represented by the ticks, are the Integers. And the Integers themselves are are composed of Natural numbers, which are your regular ‘counting numbers’ (0, 1 apple, 2 apples, 3 apples) and their negatives.
Of course, if there are Rational numbers, we must also have Irrational numbers. Irrational numbers cannot be expressed as the ratio of two integers, and so their decimal forms extend forever without repeating. Square roots are sometimes irrational (such as the square root of 2), as well as pi and e (if you haven’t already, you will learn about these special numbers later).
So then, in a nutshell, what is a Real Number? They are a set of numbers that can be broken down into a few categories. Rational numbers are all numbers that can be represented as a simple fraction or repeating decimal. Irrational numbers are all numbers whose decimals continue forever without repeating. Together, the Rational and Irrational numbers fill in our number line completely, and form the set of Real Numbers.
Hopefully this makes sense and answers the question that so many students arrive here looking for: “What are Real Numbers?” Also, I hope it gives you a deeper understanding of the numbers that you have always known how to work with anyways!

