Follow along through my example and you will hopefully be able to see what I mean.

Let’s consider the equation x² + 7x + 10 = 0

First, we can identify that there is an x² term (with a non-zero coefficient… 1), so we can say that it is a quadratic equation.

To solve a quadratic equation, we want to determine the roots, or what values make the equation true. To help us to achieve this, we want to rearrange the left side so that it is a product of two terms (or expressions). In this way, we can say that “something times something equals zero”. And since we need one of those “somethings” to be zero if the product is zero, we essentially break this down to “something #1 = 0” and “something #2 = 0”, and by solving these two simpler equations, we will arrive at our roots. So, continuing with our example then, let’s factor it. Review my post on methods of factoring if you need a bit of a refresher!

x2 + 7x + 10 = 0
(x + 2)(x + 5) = 0

This is what we’re looking for: two expressions multiplied together to give zero. Now, we have two equations to work with to find our roots of the quadratic equation. Rewriting, this gives us:

x + 2 = 0 and x + 5 = 0

And quite obviously, these can be solved to show that x = (–2) and (–5). And since we followed that whole process, we can consider these two values to be roots of our original quadratic equation. However, it is VERY IMPORTANT to substitute these values back into the original equation to check! With these values, we can show that:

(–2)2 + 7(–2) + 10 = 0
4 – 14 + 10 = 0…….. this is true. So –2 is for sure one of the roots. I’ll leave –5 for you to verify on your own.

If you find a question and proceed all the way through to find the roots, and you go and plug them back into the original equation, if one of the roots does NOT satisfy the equation, you cannot count it as one of the roots. This sometimes happens when you have an expression in a denominator (eg. (x – 2)), and if you determine through the above steps that your expression gives you a root of 2, by plugging this into your original equation, specifically into the denominator, the denominator will equal 0 and cause the expression to be undefined. Therefore, this root does not satisfy the original equation and you just ignore it.

I hope this has helped to explain the process you need to follow to solve quadratic equations. With practice, they will become second nature. However, despite all of the work required, sometimes it just is not practical or apparent how to factor your quadratic equation. In these cases, you would likely want to rely on the use of the quadratic formula, which I will go over in a future post to explain what it is and how it works. Let me know if this makes sense or if you’d like anything more added.

When most students hear “quadratic equation,” they usually get anxious because quite often this means having to work with the quadratic formula. This formula is more complicated than most that you have probably encountered up to this point, but factoring quadratics doesn’t always rely on the quadratic formula! In fact, they can be quite simple! A quadratic equation isn’t just “something that needs the quadratic formula” to solve it. Quite simply, a quadratic equation is just an equation that can written in this form:

ax² + bx + c = 0 where a, b, and c are real numbers and a does not equal 0

See? That doesn’t sound so bad. The KEY is that the “a” value is not 0. b and c can be, but not a. You need to have the x² term.

Remember, “solving an equation” means to find the roots or solution… or, what makes the expression true? To do this with quadratic equations, we rely on the property that says “two terms multiplied = 0 only if one or both of those terms is 0.” Remember this property! It is key to the quadratic factoring method. If we combine this property with our ‘grouping’ factoring method, you will see how this all comes together.

For my first example of using the quadratic formula to find the roots of a quadratic equation, let’s keep it simple.

x² – 2x –3 = 0

Comparing this to the standard form of a quadratic equation, ax² + bx + c = 0, we can equate the letter coefficients to the values provided. That is, we can say that a = 1, b = (–2), c = (–3). Now, we can simply substitute these values into the quadratic formula:

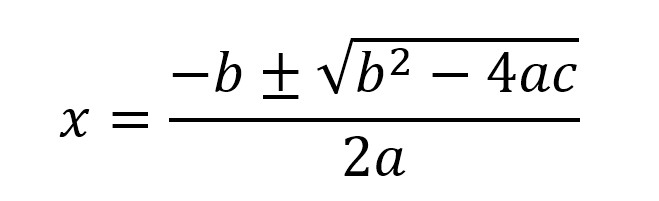
So, we have:

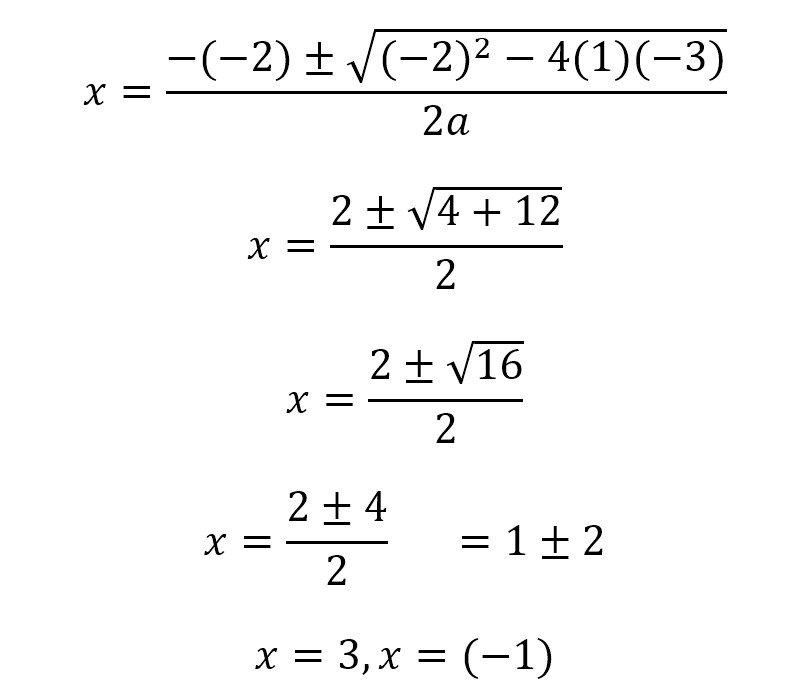
If you follow along with the arithmetic, you can see that we’ve solved the quadratic formula to show that the roots of the given equation are x = 3 and x = (–1).

Now, remember that I said in a previous lesson that you have to check your answers! Substitute these values back into the original equation, and you will find that they do indeed satisfy the equation. So, these are the correct roots!

Of course, you may have noticed that this question didn’t actually require the quadratic formula to solve for the roots. The quadratic formula worked well and got us the answer, but as you saw, it required a bit of work. And more work means more opportunity to make a mistake! You may have noticed that there was actually a faster way of solving the question. If you noticed that you could reduce the question down to (x – 3)(x + 1) = 0, you could simply let each set of brackets equal zero, and then find again that x = 3 and x = (–1) are the correct solutions.

Let’s try another one, adding some more of the previous math concepts I’ve gone over.

Using the quadratic formula, find the roots of:

2x³ + 3x² = 4x

It’s looks a little more complicated than the last one, huh? It has higher order exponents, and it doesn’t immediately look like a quadratic equation, as the first example did. However, with a little bit of arithmetic, and using your skills from the math concepts I explained in my post about factoring, it will begin to look a bit more familiar and workable.

So then, apply grouping techniques to our question. Let’s bring everything to one side first though. Recall that the standard form of a quadratic equation equals zero.

2x³ + 3x² = 4x
2x³ + 3x² – 4x = 0
x(2x² + 3x – 4) = 0

Looks a little better now, right? Maybe, something that might fit into the quadratic formula? Recall that the roots, or solutions, are any values of x that make the expression true. So, what we have derived up to this point is a product of two expressions that equals zero, and therefore the roots will be whatever values of x cause each part of the product to equal zero. The first (potential) root is obvious, from the first of the two expressions in the product: x = 0. (Substitute 0 back into the original equation to verify this is a correct root!) The second part, 2x² + 3x – 4, will require more work, and if we let it equal zero, you can see that it will fit into the quadratic formula perfectly.

To prepare for the quadratic formula, we need to identify our a, b, and c values. They are: a = 2, b = 3, and c = (–4). Now, we just substitute into the formula, do the math, and come up with our root(s) for this part of the question!

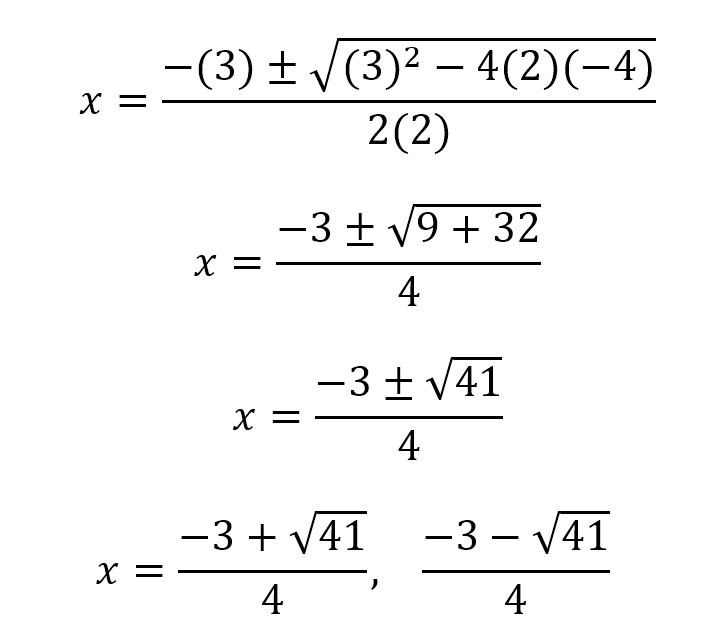
So, these are our answers for the two roots to the quadratic expression part of our original question. These are the radical forms of the solutions, so they look way more complicated. But, often the quadratic formula doesn’t reduce all the way down to a nice, round number and you will be left with something like this. The last thing you have to do is substitute them back into the original question to verify the roots are true, and that is it! Of course, when you write your answers down, make sure you remember to include the roots from the first part of the question, i.e. the part we created by grouping and solved for x = 0.

That last question goes over a lot of math concepts and is definitely comparable to some of the more complicated math questions you may find in your homework or on exams. Review and study it and make sure you understand it. I’ll post another example as well soon, if anyone needs some more examples of using the quadratic formula.

However, as I said, not all quadratic equations can be solved this way. Sometimes, they are already expressed in a simplest form, or further manipulations just make things messier. In these cases, you can use The Quadratic Formula to solve for the roots of the equations. At first glace, the quadratic formula looks like a beast of a formula to use, and even harder to memorize! But, trust me… commit this formula to memory and learn how to use it, and solving quadratic equations will become so easy for you!

So, what is the Quadratic Formula?

I will go over how to solve it, but first, the it looks like this:

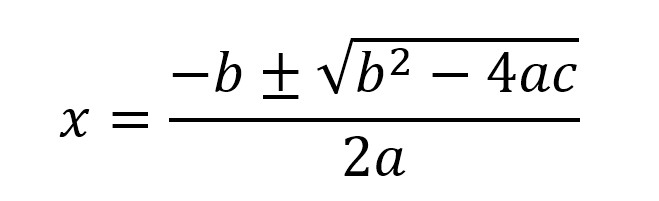
You can use this for any quadratic expression of the form ax² + bx + c = 0, where “a” does not equal zero. (If you think about this condition, you can see that if a = 0, then there is no x² term at all, and you are left with a linear equation or something of a higher order. Also, if a = 0, the quadratic formula then has 2(0) in the denominator, which equals 0 and causes the whole expression to be undefined. So, hopefully that short explanation will help you to remember that if a = 0, you cannot use the quadratic formula!)

Working through the math of the quadratic formula isn’t as difficult as you may think. To start, all you do is arrange your question into the form of ax² + bx + c = 0, and then you can easily identify the coefficients for a, b, and c. Then, you simply substitute those values into the quadratic formula, and do the math. One thing to draw your attention to though is the “plus/minus” sign. Basically, the quadratic formula is really TWO formulas, one with a “-b + √…..” and one with “-b – √…..” These two formulas are what give you your two roots.

You will study this more in the future, but for now you may find it interesting that a quadratic equation, i.e. an equation with an x² term, defines a parabola. The equation of all parabolas have x² as the highest order exponent. As a result, you can imagine that a parabola drawn on an X-Y graph will cross the x-axis twice (at the most). These are the roots or solutions of the equation, and so that is why you cannot have more than 2 roots. Similarly, you can figure out why there may be 1 or even 0 roots, depending on where the parabola is located on the graph.

So, now that you know the answer to ‘what is the quadratic formula,’ next I will show you how to use it. Examples coming in my next post.

To start, I will explain first-degree equations in one variable. Quite simply, a first-degree equation is one in which there is only one variable. In general, a first-degree or linear equation has the form:

ax + b = 0, where a and b are real numbers and a does not equal 0

I’m sure you are extremely familiar with this type of equation, though you may not know it by this name. An example of a linear equation would be something like 3x – 12 = 0. Undoubtedly, you can easily see that this equation is true when x = 4. However, it is good to realize that the expression is neither true nor false until you substitute in a value for the variable. Any value that makes the expression correct is called a solution or root of the equation. To further classify this equation, 3x – 12 = 0 is also called a conditional equation, in that it is only true for certain values.

When you have two expressions that have the same solution (or root), these are called equivalent equations. Again, I’m sure you are familiar with the concept, but probably unfamiliar with this name. When you have a first-degree equation in one variable, the general strategy that you typically employ is to express the equation equal to a series of equivalent equations, which you manipulate until you can reduce everything down to the solution to the equation.

The rules for generating equivalent equations are simple and intuitive:

• You can add or subtract the same value from both sides of the equation. (A corollary to this is that you can add AND subtract the same value on one side, without changing the other… since adding x and then subtracting x means you really have done nothing!)
• You can multiply or divide each side of the equation by the same value.
• You can simplify one side of the equation without affecting the other side of the equation.

I think these rules are fairly self-explanatory, so I’m not going to bother going into any examples to demonstrate them.

When you have arrived at your solution / root of your equation, it is ALWAYS smart to take that value and substitute it into the original expression to verify that it is indeed true. It always amazes me how many people arrive at incorrect answers and leave it at that, when a simple review and check can either tell you that you are correct, or your answer needs more work. ALWAYS REMEMBER TO CHECK YOUR ANSWERS!

By checking your answers by substituting the solution into the equation, you sometimes will determine that the solution you have found CANNOT be true, in which case your solution is called an extraneous root. An example of this would be where, when checking your solution, you determine that you have a 0 on the bottom of a fraction (the denominator). A fraction with a zero in the denominator is undefined, and so you can conclude that the root you determined does not satisfy your equation. Extraneous roots may develop especially if you use rule number 2 above, but you multiply both sides by an EXPRESSION rather than a single number. (eg. you multiply both sides by (x + 2))

That is all I am going to say about how to solve equations for now, especially the first-order equations (or linear equations). I will continue in my next post with a discussion of solving quadratic equations.

First of all, a high level, general definition is needed. What does factoring mean? Factoring means to simplify a mathematic expression by writing the expression as a product of two or more values or expressions. For example, if you factor the expression 12x + 4, you get (4)(3x+1). This is an example of “factoring out” the 4. You will see this phrase “factoring out” very commonly when dealing with these types of problems.

Several factoring techniques are available to you to help you find factors, depending on the question. These are some techniques you can use. You can memorize these factoring tricks and shortcuts and you will save a lot of time with your math work! If you do lots of practice with factoring games or math worksheets, you will become good at these and be able to find factors very quickly.

Common factor

In an expression composed of multiple terms, try to identify if there is one number/variable that is a common factor to each term. Then, after factoring out the common factor, you can rewrite the expression to show multiplying that common factor by the remaining terms.

12x + 4 = (4)(3x + 1)
5x³ + 10x² + 25x = (5x)(x2 + 2x + 5)

Difference of squares

Wherever you see a difference of two terms that are perfect squares (either something like x² or 25), you can apply this technique for factoring a difference of two squares. This actually is the same as one of the rules for special polynomial products. In fact, factoring special products follow the same rules you would use to find factors anywhere else. Factoring squares is actually quite simple:

x² – a² = (x – a)(x + a)

You reduce the terms to their square root value, and remember to put one ‘+’ and one ‘-’. Easy as that.

Difference of cubes / Sum of cubes

Factoring perfect cubes (or factoring cubics) is a little trickier, but they follow a strict form that you can memorize and use easily to find factors. Depending on if you are subtracting perfect cubes or adding perfect cubes, you will use the appropriate formula:

x³ – a³ = (x – a)(x² + ax + a²)
x³ + a³ = (x + a)(x² – ax + a²)

They have the same basic form, you just have to pay attention to the signs. Don’t mix them up!

Grouping

This is an extension of the common factor method described above. The only difference is that the common factor doesn’t have to be common with EVERY term. Group things together, and factor within the groups. Take this example and you should see what I mean:

x³ – x² + x –1
= (x³ – x²) + (x –1)
= x²(x – 1) + (x – 1)
= (x – 1) (x² + 1)

Study that example and it should be fairly self-explanatory how I used grouping to find factors here.

Trial and Error

Sometimes, there aren’t any obvious factoring tricks or factoring techniques that you can apply to help you solve your question. Unfortunately, in these situations, you must resort to trial and error. Sometimes you can figure out the numbers that are involved, but you need to test out the signs to get it right. I can’t really say anything about this technique except to have patience and keep trying.

I will revisit this post shortly to put up a remark about factoring quadratic equations. I will also go over AC method factoring. The AC method of factoring is a factoring method or factoring trick you can use to help you factor expressions in the form of ax² + bx + c (trinomial).

First, let’s consider the derivative in terms of rate of change. And to do that, let’s talk about velocity. We know that velocity is equal to distance per unit time. However, that is a very general description of it. If you drive from your home to the grocery store on the other side of town, you can do the math by dividing your total distance travelled by the time it took you to get there, but what does this number tell you? It actually tells you the average velocity of your trip. Think about it. You had to stop for red lights, stop signs, pedestrians. Maybe you sped up to pass a slow driver. Don’t forget about the actual acceleration of your car from a standstill, and then the deceleration whenever you needed to stop. All of this factors into the calculation of your average velocity, which is simply how far you go in a measured amount of time.

Now, let’s consider how to calculate the average velocity of your car between your home and the first stop sign, while on your way to the grocery store. You no longer have several stops to deal with. You get in your car, accelerate, then as you approach the stop sign, you decelerate to a stop. Your average velocity is calculated from a much shorter interval, and will have much less variation to it. So, your average velocity will be more representative of your actual velocity at any given time.

To extend this demonstration even further, let’s consider the small portion of your trip that is measured between two street lights 10 meters apart that you pass while you are in full motion. That is, let’s assume that we want to measure the velocity without having to calculate stop signs, etc. Our time interval for the measurement is much smaller, and calculating the velocity by dividing the distance by the time it takes to go from one light to the other is even more representative of your velocity at any point between them.

What I am trying to demonstrate is the concept of instantaneous velocity. If you shrink down your time interval of measurement infinitesimally, the two time points approach each other at a single point, and so the average velocity between the two super close points approaches the instantaneous velocity of the single point.

Graphically, this is essentially the same thing you do when you calculate the slope of a tangent line to a curve. You pick two lines on the curve and calculate the slope of the line between them, and then you use limits to make the points get closer and closer together until they are almost the same, and the slope of the line connecting those two infinitely close points is the tangent. (Check out my previous post about using limits to find tangents if you’d like a refresher of this topic)

Because this type of limit occurs so frequently in maths, science, and engineering, it is given the special name of “derivative,” and you calculate derivatives through the process of “differentiation.” So, one interpretation of the derivative is an expression of the instantaneous rate of change (velocity) at a particular point on the curve – a large derivative corresponds to a high rate of change (a steep curve), and conversely a small derivative corresponds to a low rate of change (a relatively flat curve). As a specific example, if you actually have a graph of position (displacement) of an object vs. time, the derivative of the curve at any time point represents the velocity of that object at that specific time. This may take a little practice to become comfortable with the concept, but suffice it to say at this point that learning how to use derivatives is incredibly important to be able to work out more complex concepts relatively easily.

Let’s look at this now in the more formal terms of mathematical symbols and equations. Consider any curve y = f(x).

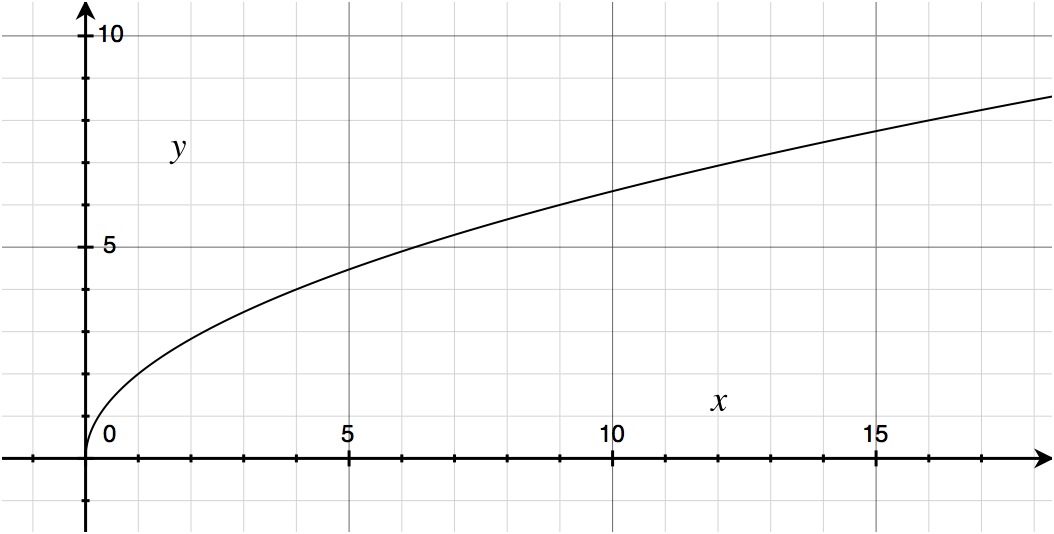
Now, let us identify the point P on the curve f(x) for when x = a. That is to say, the point (a, f(a)).

Now, let’s go a step further, and identify a point Q that is h units away from a on the x-axis. If it is h units away from a, we can call it “a + h”. (If this is confusing, think about it with numbers instead. Start at, say, x = 3 (instead of a). Now we want to know what is going on 5 units (instead of h) away from x = 3. In other words, we have 3, and we have 3 + 5.) As such, we can therefore identify a point Q ((a + h), f(a + h)).

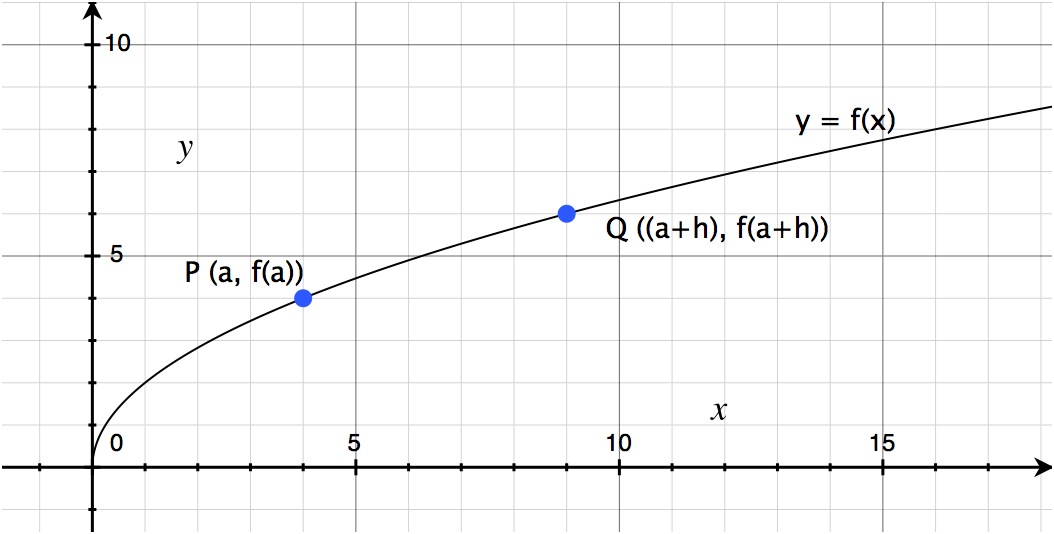
Now that we have two arbitrary points, let’s determine the slope of the straight line that would connect the two. We can use the same slope formula that we always use, slope = rise/run, but substitute in our variables that we identified above. So, we have:

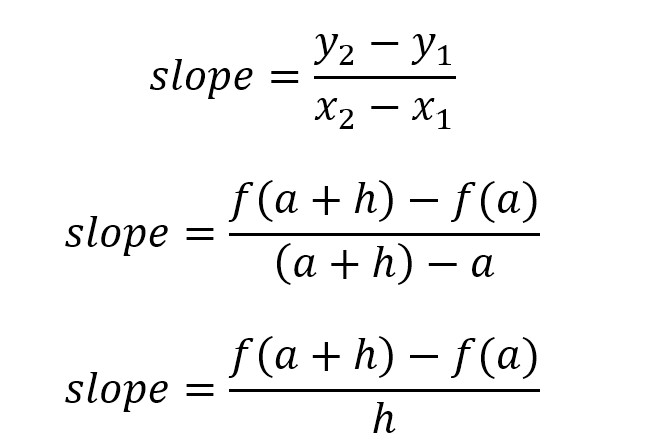
Now, imagine that the distance h between the two points is getting smaller and smaller. Or in other words, consider the case of when h approaches 0. By doing this, we calculate the slope of the line connecting two infinitesimally close points – which means that we are actually approaching the slope of the tangent line to the curve at point a. In this case, we would express this slope as a limit in the following way, which actually corresponds to the definition of the derivative of a function f at a number a. The derivative is given the special symbol f’(x), and we say “f prime x”, and we express it like this:

Another way of expressing this can be found if we recognize that a + h is really just any x value. So, we can say x = a + h (and by extension, h = x – a), and modify the above derivative definition accordingly:

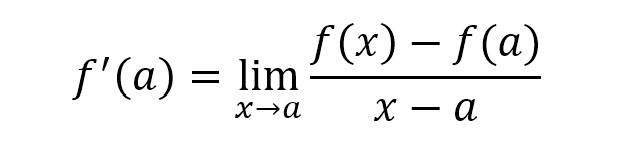
With this modified equation, it actually becomes a matter of arithmetic to determine the slope at a point. Here is an example of a kind of question that you will see:

“Find the derivative (or, determine the slope of the tangent) of the function f(x) = x2 – 4 at a number a.”

To do this, write the provided equation into the definition, and reduce until you have an answer. Notice below how I combine terms and recognize the identity of a difference of squares.

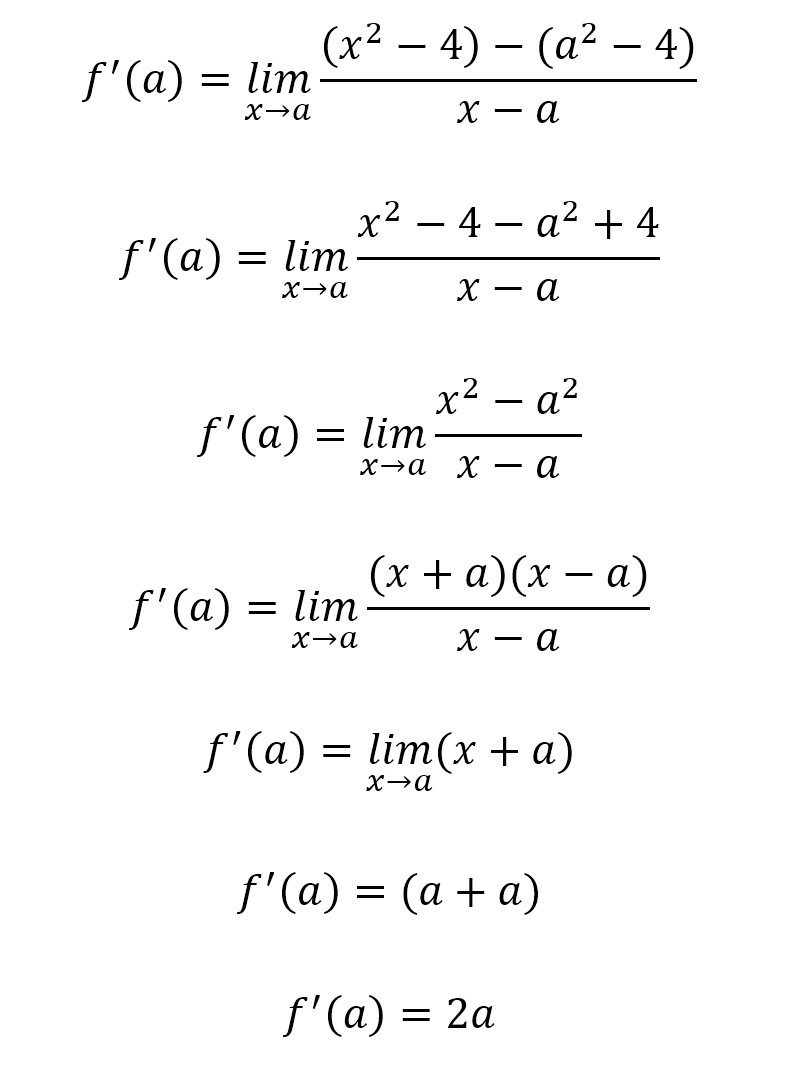
What this final result tells you is that for our curve, f(x) = x2 – 4, at any number a along it, the slope of the tangent (AKA, the derivative at that point) is equal to the term 2a. Graph it out and try with several values to convince yourself that it’s true! Consider when x = 5. You can determine from the original equation that we have the point (5, 21). At this point on the curve, the slope of the tangent equals 2 x 5 = 10.

Going back to the definition of the derivative that I gave above, you can also apply the concept of point-slope form to it to get a different way of seeing it. Letting y = f(x), you can rearrange the definition as follows, by simple reorganization of the terms:

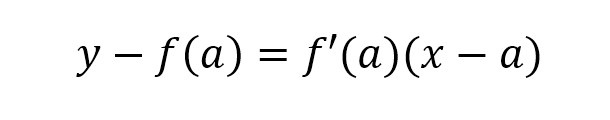
Here’s a more visual exercise that you may soon encounter.

“If you are provided a graph of a function f(x) – not necessarily the equation – sketch out what the graph of the derivative f’(x) would look like.”

When you actually have the numbers and equation, this becomes much easier… assuming you know how to easily recognize derivatives from the original equations. However, if provided ONLY the picture of the curve, this becomes a bit more abstract, but not really that challenging. It DOES require you to understand the concept of derivatives and rate of change though. Here is why. Take some random curve that you can draw. Any curve will do for this exercise:

The key is rate of change. We have seen that slope is equal to rate of change, so we want to pay particular attention to the slope at several points. And the easiest points to notice are those where the slope is equal to 0. These are all the peaks and valleys of the curve. What I have done next is highlight with red bars all of the zero-slopes:

Now, to proceed with sketching the graph of the derivative f‘(x) vs x, you can start by plotting the points where f’(x) is equal to zero. From there you can then go on to say where the curve of f(x) has a positive, increasing slope, and then sketch that into your f‘(x) graph accordingly. Similarly, decreasing slopes on the f(x) curve will be negative values on the f’(x) curve. For the sake of this exercise, don’t worry so much about how high or low the slopes are. Just focus on whether they are positive or negative at the various parts of the graph. I have gone ahead and plotted out the actual curve of the derivative below in green, alongside the original curve of f(x). You can see that the f‘(x) curve crosses zero wherever the curve for f(x) has peaks or valleys, and the steeper the f(x) curve, the more extreme the f’(x) curve at that same point.

Of course, having a mathematical definition wouldn’t be any fun if there were no conditions or rules associated with it – and the definition of derivatives is no exception. One such rule states that a function is differentiable at a point a if the derivative f’(a) exists. Seems intuitive enough. If a derivative at a point exists, then the base function is differentiable at that point. Probably one of those rules that doesn’t really even need to be said.

I’m not going to graph this one out, but it is for you to think on. Consider the case of f(x) = |x|. Where is it differentiable?

If you consider the derivative of the left hand side, it equals –1. The f‘(x) on the right hand side is 1. This function then is obviously differentiable when x < 0, and when x > 0. But what about when x = 0? In this case, since the right hand limit approaches 1 as x approaches 0 from the right, and the left hand limit approaches –1 as x approaches 0 from the left, one must conclude that f’(0) does not exist because both of the one-sided limits approach different numbers.

An extension of this example actually describes a second rule for limits: if f’(a) exists, then the function f(x) is continuous as a. Recall that continuity of a curve is based on the notion that as you approach a point from both the left and the right, the limit of each side approaches the same value. In the example above, approaching 0 from either side resulted in different limits, and hence the graph is not continuous at 0.

Keep this in mind as you see various graphs of functions. Curves that have a sharp point will not be differentiable at the point, for the reason given above. Similarly, discontinuous curves (i.e. curves with gaps in them) will not have a derivative at the break point either because the one-sided limits do not agree. If f(x) is not continuous as point a, then f’(a) does not exist. A third condition to watch out for is where a graph has a vertical tangent line, in which case the slope is infinite.

Now, I’m going to wrap up this mammoth of a math post with something a bit easier to talk about: notation of derivatives. I have already described a few ways to express these values. I talked about expressing them as limits, and using infinitesimally smaller intervals, and that is a good way to work through them. Symbolically, I said that you can write f’(x) to denote the derivative of the functions f(x). This will likely be the easiest way for you to use it and to recognize it, though here are a few others that mean the same thing:

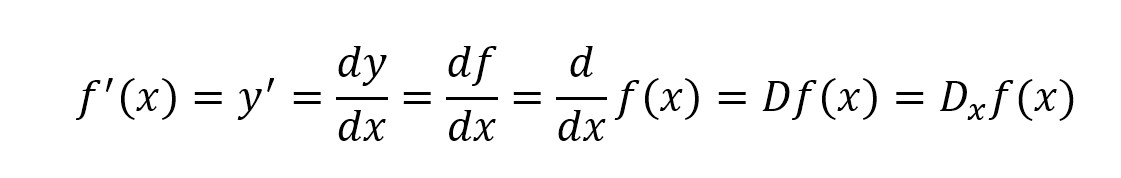
Each of these terms means the exact same thing. In particular, the D and d/dx are specifically called the differentiation operators, and you can see they have a few variations. Similarly, dy/dx is symbolic of derivatives for historical reasons. Read up on Gottfried Wilhelm Leibniz to learn more about the origins of calculus, where you will see that he introduced this way of representing it. Sometimes, you may see dy/dx referred to as “Leibniz notation.”

And with that final tidbit of mathematical goodness, I am going to end this post. I intend to follow this with another post in the near future that introduces differentiation methods and strategies. Much like the exponent rules, there are also several differentiation rules, and I hope to be able to explain them for you as well. If you have made it to this point of my post, thanks for reading, and please be sure to click the Facebook Like button below or at the top and share with your friends!

As you look at these logarithm rules, keep in mind that by convention, if you write logs without the subscript number to indicate their base, it is assumed that you are dealing in base–10. For simplicity, this is the convention that I am going to use in this post, though these rules certainly apply when dealing with logs of other bases.

With that intro out of the way, let’s get to it.

The first law of logarithms is the product rule. If you are familiar with the product rule of exponents, then this logarithm law should be a piece of cake for you. Where the exponent rule says that when multiplying exponential expressions with the same base, you simply add the exponents, this same thing applies when multiplying logarithms of the same base. Therefore, the rule states that the logarithm of a product is equal to the sum of the logarithms.

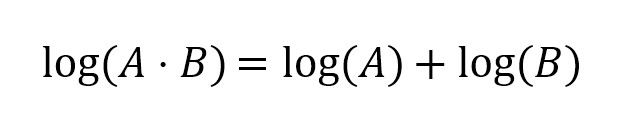
This rule is very commonly used, and it is important to recognize that you can use it in either direction. That is, the logarithm of a product converts to a sum of logarithms, and vice versa.

The next logarithmic law is the quotient rule. Again, this law can easily be derived by applying your knowledge of the exponent quotient rule (though I will leave that for you as an exercise). However, it does appear to look different. This rule states that the logarithm of a quotient is equal to the logarithm of the numerator minus the logarithm of the denominator. Sounds like a mouthful, but the expression is probably much simpler to understand.

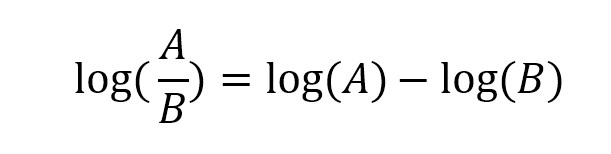
Again, watch for opportunities to use this relationship in either direction!

The third law of logs is the power rule. This one is surprisingly simple to remember, and again can be found by manipulating exponent and logarithm laws. Quite simply, this law says that when you have a logarithm of an exponential expression, the exponent can be “brought out” of the log and used as a coefficient for the log.

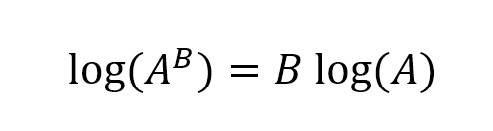
The last of the rules of logarithms that I’m going to discuss here today is called the base change rule. Recall that I stated above that all of my examples that I’ve used so far in this post use the convention of an assumed base–10. If I wanted to change my expression to utilize a different base, this rule helps us to do that. So then, if I have my log in some base of a number, and I want to express this in terms of a different base, I simply take the log in my new base of the original number and divide that by the log in my new base of the original base. Sounds wordy, but again, a picture is worth a thousand words:

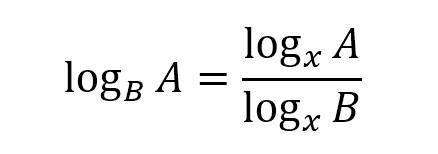
Here, my original base is B, and my new base that I want to express things in is X.

That is all I have to say about the rules of logarithms in this short introduction to them. They are fairly straight forward themselves, though can be used in very complex equations. I will try to do a separate post soon outlining some examples of all of these rules, though I do think that the general forms that I’ve noted above are pretty self-explanatory.

If you are interested in learning more about logarithms, there is a much more thorough summary of logarithms at the Learning and Teaching Math blog, which I highly recommend (for this and other math topics!)

Consider a curve on a graph – any curve will do. Now, pick a point on the graph, and draw a tangent to the graph at that point. Recall that a tangent to a curve is a line which touches the curve at only a single point. This tangent line is a representation of the derivative of that curve at that particular point on the curve. Naturally, every point on your curve will have its own tangent line. Here is a rough example, where I have generated some curve, and the red lines indicate the tangent lines to the curve at the points where the lines touch the curve:

By using the derivative of the equation of your curve, you are able to precisely calculate what the tangent line will look like at any point on your curve. In fact, the derivative of your equation will very likely be another equation, and you can graph this new equation out to see the graph of the derivative, which itself can be interpreted and used in many ways in calculus. You can even find the derivative of the derivative, etc.

Let’s look at a more practical example to hopefully convey the usefulness and significance of calculating derivatives.

If we consider two points A and B, and we know it takes some time to travel from A to B, we can calculate the “average” velocity of this trip by dividing the distance between the two points by the time taken to travel between them. This should be a familiar notion. However, consider this: if we are driving in a car from home to the market, then we do not have the same velocity for the entire trip. We have to start accelerating from being parked, and then decelerate when we come to stop signs, and then accelerate again, and decelerate to a stop when we reach our destination. You can calculate the average velocity of this trip in the way I mentioned above, but the “instantaneous” velocity at any particular point of the trip might be what you are more interested in. How might you go about doing this? Find the derivative! A graph of our trip, where we plot distance travelled against time, might be useful in this case. We can determine the tangents to this curve at any particular points. We know from previous graphing experience that these tangent lines have a slope of rise over run, and distance over time (the y and x axes on this graph) is velocity. So therefore, the tangent line (specifically, its slope) at any particular point on our curve actually represents the instantaneous velocity at that point. So, we can find out exactly how fast we are going at any point of our trip. We just demonstrated this graphically, but by calculating the derivative of our “trip equation” means that we can calculate how fast we were going any any point without actually graphing our equation at all!

This is just an introduction to the concept of derivatives in calculus, but in future posts, I’ll go into more details so that hopefully you can become more familiar with them. The concept might be new and different, but once you begin working with them, you will see that they are not that difficult to work with at all.

The decimal numeral system is the counting system most widely used around the world. You may also hear of it referred to as a base ten system. In fact, this is where the word “decimal” comes from; Latin “decem” means ten. When we say it is a base ten system, that means that each digit position has ten possibilities, or more specifically, can be any of the digits 0 – 9. So, if we count out 15 apples, we know that this breaks down to a 5 in the ones column and a 1 in the tens column, and so we automatically equate this as 1 times ten and 5 times one to make 15. This also shows the importance of each position in the number. In a base ten system, there is a column for ones, tens, hundreds, thousands, etc. All of this is probably very familiar already, so I’m not going to go into any further details of the decimal system.

Now, let’s look at the binary numeral system. While the decimal system is the most commonly used system, probably a very high percentage of people are at least aware of the binary system, though they probably don’t fully understand it. The binary system is a base two system, usually denoted as 0 and 1. In fact, the reason why binary is historically associated with computer programming is because this 0 and 1 can physically be represented in computer circuitry as being “open” and “closed” states – way more than I’m going to explain here, but interesting nonetheless. If we consider a base two system, we quickly see that counting is an entirely different experience. Unlike the base ten system with ones, tens, hundreds, since the base two system only uses two digits, instead it has digit positions for ones, twos, fours, eights, sixteens, etc. Each position is a factor of 2 greater than the position to its right, much like in base ten where each position is a factor of 10 greater than the position on its right.

Let’s count apples again to see binary counting in action:

• 0 apples = 0 in binary
• 1 apple = 1 in binary
• 2 apples = 10 in binary
• 3 apples = 11 in binary
• 4 apples = 100 in binary
• 5 apples = 101 in binary
• 6 apples = 110 in binary
• 7 apples = 111 in binary (= 1 one + 1 two + 1 four)
• 8 apples = 1000 in binary

The important thing to realize is that physically, we have the same quantity of apples, regardless of the numeral system we are using to represent them. So to speak of 8 (base ten) apples or 1000 (base two) apples, we are really talking about the same thing.

We can apply these counting concepts to the other numeral systems as well. Let’s look at the octal numeral system first. As you can probably tell from its name, octal is a base eight system. So, each number position can have any of the digits 0–7, and the labelling of the positions goes up by a factor of 8 for each position. So, we have ones, eights, sixty-fours, five hundred twelves, etc. Let’s look at some apple quantities again, but in octal this time. You can figure out what 0–7 apples looks like in octal notation, but what about 8 or higher:

• 7 apples = 7 in octal
• 8 apples = 10 in octal
• 9 apples = 11 in octal (= 1 one + 1 eight)
• 10 apples = 12 in octal
• 11 apples = 13 in octal (= 3 ones + 1 eight)
• …
• 20 apples = 24 in octal
• 24 apples = 30 in octal
• 30 apples = 36 in octal (= 6 ones + 3 eights)
• …
• 100 apples = 144 in octal (=4 ones + 4 eights + 1 sixty-four)
• …
• 750 apples = 1356 in octal (= 1 five hundred twelve + 3 sixty-fours + 5 eights + 6 ones)

Finally, let’s look at the hexadecimal number system. This system, also used frequently in computer programming, is a base sixteen system (as can be seen by its name: hexa- mean six and deci- means ten = 6+10 = 16). This counting system may take the most work to understand, but again, it follows the same rules. In this case, however, we run into a wrinkle. So far, we have looked at decimal, binary, and octal systems. All of these utilize at least some of the familiar digits 0–9. But how do we represent a number between 10–15 by only using a single digit? We only know of these quantities as two-digit numbers! Is there anything else we might use that is only a single character, without having to make up a brand new one? This is where hexadecimal is slightly different from the others. It uses 0–9 to represent those digits, though for 10–15 it uses the letters A-F. So, to write 10 in hexadecimal, it is simply A. Similarly, 11 is B, and so on. As a result, the number positions again are larger than the previous systems, so we have ones, sixteens, two hundred fifty-sixes, four thousand ninety-sixes, etc.

So counting apples again!

• 13 apples = D apples in hex
• 15 apples = F apples in hex
• 16 apples = 10 apples in hex
• 26 apples = 1A apples in hex
• 100 apples = 64 apples in hex
• 124 apples = 7C apples in hex
• …
• 50000 apples = C350 apples in hex

By including letter characters as digits, hexadecimal may be the most challenging of these number systems to wrap your head around. The bigger the numbers get, the more complicated they may seem. However, they all follow the same concepts and aren’t too difficult to figure out, once you get used to thinking in a number system other than base ten!